检查颜色相似度的算法

Question

我正在寻找一种算法，可以比较两种 RGB 颜色并生成它们的相似度值（其中相似度意味着“与人类平均感知相似”）。

有什么想法吗？

编辑：

由于我无法再回答，我决定将我的“解决方案”作为对问题的编辑。

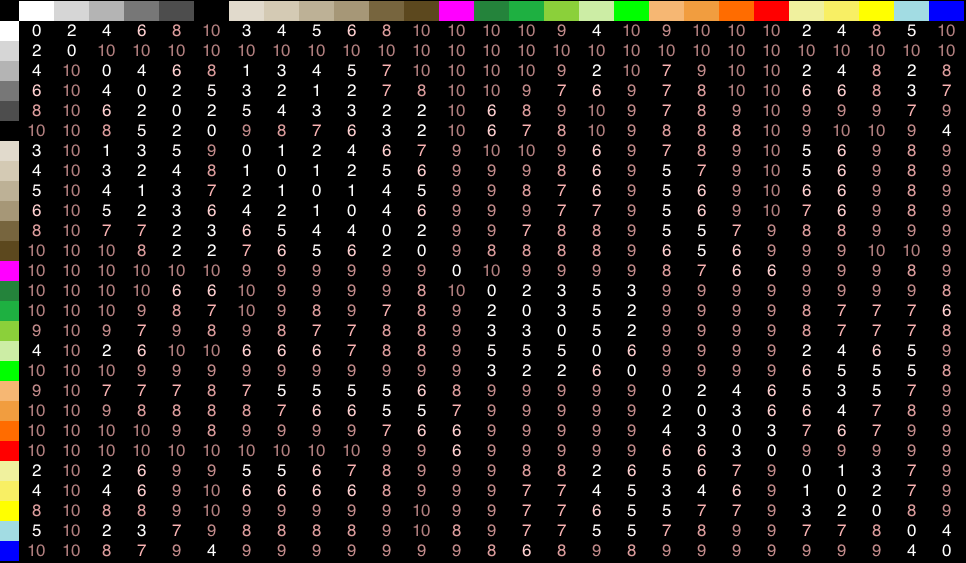
我决定在我的应用程序中使用（非常）小的真彩色子集，以便我可以自己处理颜色比较。我使用大约 30 种颜色，并在它们之间使用硬编码距离。

因为它是一个 iPhone 应用程序，所以我使用 Objective-C，其实现或多或少是一个代表下表的矩阵，它显示了颜色之间的距离。

Answer 1

RGB 距离 在 欧几里得空间与“平均人类感知”不太相似。

您可以使用 YUV 色彩空间，它考虑到了这个因素：

 |  Y' |     |  0.299     0.587    0.114   | | R |
 |  U  |  =  | -0.14713  -0.28886  0.436   | | G |
 |  V  |     |  0.615    -0.51499 -0.10001 | | B |

您还可以使用 CIE 用于此目的的色彩空间。

编辑：

我要提到YUV色彩空间是一种廉价的近似值，可以通过简单的公式计算。但它在感知上并不统一。感知均匀意味着颜色值的相同量的变化应该产生大约相同的视觉重要性的变化。
如果您需要更精确和严格的指标，您一定要考虑 CIELAB 色彩空间或其他感知统一的色彩空间空间（即使没有简单的转换公式）。

Answer 2

我建议使用 CIE94 (DeltaE-1994)，据说它可以很好地代表人类颜色感知。我在计算机视觉相关的应用程序中经常使用它，并且我对结果非常满意。

然而，执行这样的比较的计算量相当大：

1. 两种颜色的 RGB 到 XYZ
2. XYZ 到 LAB 两种颜色
3. Diff = DeltaE94(LABColor1,LABColor2)代码>

公式（伪代码）：

• 颜色转换公式：http://www.easyrgb.com/?X=MATH
• Delta-E 公式：http://www.easyrgb.com/index.php?X=DELT

Answer 3

这里有一篇关于颜色距离主题的精彩文章：
http://www.compuphase.com/cmetric.htm

如果该资源消失了作者的结论是可以使用此公式（在 C 代码中）实现两种 RGB 颜色之间距离的最佳低成本近似。

typedef struct {
   unsigned char r, g, b;
} RGB;

double ColourDistance(RGB e1, RGB e2)
{
  long rmean = ( (long)e1.r + (long)e2.r ) / 2;
  long r = (long)e1.r - (long)e2.r;
  long g = (long)e1.g - (long)e2.g;
  long b = (long)e1.b - (long)e2.b;
  return sqrt((((512+rmean)*r*r)>>8) + 4*g*g + (((767-rmean)*b*b)>>8));
}

Answer 4

人类对色度的感知比对强度的感知弱。

例如，在商业视频中，YCbCr/YPbPr 色彩空间（也称为 Y'UV）会降低色度信息的分辨率，但保留亮度 (Y)。在数字视频压缩中，例如 4:2:0 和 4:2:2，由于感知相对较弱，因此会降低色度比特率。

我相信您可以计算一个距离函数，该距离函数的优先级高于亮度 (Y)，而优先级低于色度。

此外，在低强度下，人类视觉几乎是黑白的。因此，优先级函数是非线性的，因为对于低亮度 (Y)，您对色度的权重越来越小。

更科学的公式：http://en.wikipedia.org/wiki/Color_difference

Answer 5

颜色感知不是欧几里得的。任何距离公式都既足够好，同时又很糟糕。任何基于欧几里得距离（RGB、HSV、Luv、Lab...）的测量对于相似的颜色都足够好，显示水绿色接近青色。但对于非接近值来说，它是任意的。例如，红色更接近绿色还是更接近蓝色？

来自 Charles Poynton 的颜色常见问题解答：

XYZ 和 RGB 系统相差甚远
表现出感知的一致性。
找到 XYZ 的变换
合理感知均匀的空间
在 CIE 上花费了十年或更长时间
最终没有一个系统能够
达成一致。

Answer 6

RGB 立方体中的颜色相似度通过欧几里得距离来测量（使用毕达哥拉斯公式）。

编辑：再想一想，对于大多数其他色彩空间来说也应该如此。