Question

MATLAB提供了用于计算符号导数的diff命令。 在最简单的形式中，您将要区分为diff命令的函数作为参数传递。

例如，让我们计算函数f（t）= 3t ² + 2t ^-2的导数

例子 (Example)

创建一个脚本文件并在其中键入以下代码 -

syms t
f = 3*t^2 + 2*t^(-2);
diff(f)

编译并执行上述代码时，会产生以下结果 -

ans =
6*t - 4/t^3

以下是Octave相当于上面的计算 -

pkg load symbolic
symbols
t = sym("t");
f = 3*t^2 + 2*t^(-2);
differentiate(f,t)

Octave执行代码并返回以下结果 -

ans =
   -(4.0)*t^(-3.0)+(6.0)*t

基本分化规则的验证

让我们简要说明用于区分函数的各种方程或规则并验证这些规则。 为此，我们将f'（x）表示为一阶导数，f“（x）表示二阶导数。

以下是差异化的规则 -

Rule 1

对于任何函数f和g以及任何实数a和b是函数的导数 -

h(x) = af(x) + bg(x)相对于x由下式给出 -

h'(x) = af'(x) + bg'(x)

Rule 2

sum规则表明如果f和g是两个函数，f'和g'分别是它们的导数，那么，

（ f + g)' = f' + g'

(f - g)' = f' - g'

Rule 3

product规则规定如果f和g是两个函数，f'和g'分别是它们的导数，那么，

(fg)' = f'.g + g'.f

Rule 4

quotient规则指出如果f和g是两个函数，f'和g'分别是它们的导数，那么，

(f/g)' = (f'.g - g'.f)/g ²

Rule 5

polynomial或基本幂次规则表明，如果y = f(x) = x ⁿ ，则f' = n. x ^(n-1) f' = n. x ^(n-1)

该规则的直接结果是任何常数的导数为零，即，如果y = k ，则任何常数

f' = 0

Rule 6

chain规则指出函数h(x) = f(g(x))的函数相对于x的导数是，

h'(x)= f'(g(x)).g'(x)

例子 (Example)

创建一个脚本文件并在其中键入以下代码 -

syms x
syms t
f = (x + 2)*(x^2 + 3)
der1 = diff(f)
f = (t^2 + 3)*(sqrt(t) + t^3)
der2 = diff(f)
f = (x^2 - 2*x + 1)*(3*x^3 - 5*x^2 + 2)
der3 = diff(f)
f = (2*x^2 + 3*x)/(x^3 + 1)
der4 = diff(f)
f = (x^2 + 1)^17
der5 = diff(f)
f = (t^3 + 3* t^2 + 5*t -9)^(-6)
der6 = diff(f)

运行该文件时，MATLAB显示以下结果 -

f =
   (x^2 + 3)*(x + 2)
   der1 =
   2*x*(x + 2) + x^2 + 3
f =
   (t^(1/2) + t^3)*(t^2 + 3)
   der2 =
   (t^2 + 3)*(3*t^2 + 1/(2*t^(1/2))) + 2*t*(t^(1/2) + t^3)
f =
   (x^2 - 2*x + 1)*(3*x^3 - 5*x^2 + 2)
der3 =
   (2*x - 2)*(3*x^3 - 5*x^2 + 2) - (- 9*x^2 + 10*x)*(x^2 - 2*x + 1)
f =
   (2*x^2 + 3*x)/(x^3 + 1)
der4 =
   (4*x + 3)/(x^3 + 1) - (3*x^2*(2*x^2 + 3*x))/(x^3 + 1)^2
f =
   (x^2 + 1)^17
der5 =
   34*x*(x^2 + 1)^16
f =
   1/(t^3 + 3*t^2 + 5*t - 9)^6
der6 =
   -(6*(3*t^2 + 6*t + 5))/(t^3 + 3*t^2 + 5*t - 9)^7

以下是Octave相当于上面的计算 -

pkg load symbolic
symbols
x = sym("x");
t = sym("t");
f = (x + 2)*(x^2 + 3) 
der1 = differentiate(f,x) 
f = (t^2 + 3)*(t^(1/2) + t^3) 
der2 = differentiate(f,t) 
f = (x^2 - 2*x + 1)*(3*x^3 - 5*x^2 + 2) 
der3 = differentiate(f,x) 
f = (2*x^2 + 3*x)/(x^3 + 1) 
der4 = differentiate(f,x) 
f = (x^2 + 1)^17 
der5 = differentiate(f,x) 
f = (t^3 + 3* t^2 + 5*t -9)^(-6) 
der6 = differentiate(f,t)

Octave执行代码并返回以下结果 -

f =
(2.0+x)*(3.0+x^(2.0))
der1 =
3.0+x^(2.0)+(2.0)*(2.0+x)*x
f =
(t^(3.0)+sqrt(t))*(3.0+t^(2.0))
der2 =
(2.0)*(t^(3.0)+sqrt(t))*t+((3.0)*t^(2.0)+(0.5)*t^(-0.5))*(3.0+t^(2.0))
f =
(1.0+x^(2.0)-(2.0)*x)*(2.0-(5.0)*x^(2.0)+(3.0)*x^(3.0))
der3 =
(-2.0+(2.0)*x)*(2.0-(5.0)*x^(2.0)+(3.0)*x^(3.0))+((9.0)*x^(2.0)-(10.0)*x)*(1.0+x^(2.0)-(2.0)*x)
f =
(1.0+x^(3.0))^(-1)*((2.0)*x^(2.0)+(3.0)*x)
der4 =
(1.0+x^(3.0))^(-1)*(3.0+(4.0)*x)-(3.0)*(1.0+x^(3.0))^(-2)*x^(2.0)*((2.0)*x^(2.0)+(3.0)*x)
f =
(1.0+x^(2.0))^(17.0)
der5 =
(34.0)*(1.0+x^(2.0))^(16.0)*x
f =
(-9.0+(3.0)*t^(2.0)+t^(3.0)+(5.0)*t)^(-6.0)
der6 =
-(6.0)*(-9.0+(3.0)*t^(2.0)+t^(3.0)+(5.0)*t)^(-7.0)*(5.0+(3.0)*t^(2.0)+(6.0)*t)

指数，对数和三角函数的导数

下表提供了常用指数函数，对数函数和三角函数的导数 -

功能	衍生物
c ax	c ax .ln ca（ln是自然对数）
e x	e x
ln x	1/x
ln c x	1/x.ln c
x x	x x 。（1 + ln x）
sin(x)	cos(x)
cos(x)	-sin(x)
tan(x)	sec 2 （x），或1/cos 2 （x），或1 + tan 2 （x）
cot(x)	-csc 2 （x），或-1/sin 2 （x），或 - （1 + cot 2 （x））
sec(x)	sec(x).tan(x)
csc(x)	-csc(x).cot(x)

例子 (Example)

创建一个脚本文件并在其中键入以下代码 -

syms x
y = exp(x)
diff(y)
y = x^9
diff(y)
y = sin(x)
diff(y)
y = tan(x)
diff(y)
y = cos(x)
diff(y)
y = log(x)
diff(y)
y = log10(x)
diff(y)
y = sin(x)^2
diff(y)
y = cos(3*x^2 + 2*x + 1)
diff(y)
y = exp(x)/sin(x)
diff(y)

运行该文件时，MATLAB显示以下结果 -

y =
   exp(x)
   ans =
   exp(x)
y =
   x^9
   ans =
   9*x^8
y =
   sin(x)
   ans =
   cos(x)
y =
   tan(x)
   ans =
   tan(x)^2 + 1
y =
   cos(x)
   ans =
   -sin(x)
y =
   log(x)
   ans =
   1/x
y =
   log(x)/log(10)
   ans =
   1/(x*log(10))
y =
   sin(x)^2
   ans =
   2*cos(x)*sin(x)
y =
   cos(3*x^2 + 2*x + 1)
   ans =
   -sin(3*x^2 + 2*x + 1)*(6*x + 2)
y =
   exp(x)/sin(x)
   ans =
   exp(x)/sin(x) - (exp(x)*cos(x))/sin(x)^2

以下是Octave相当于上面的计算 -

pkg load symbolic
symbols
x = sym("x");
y = Exp(x)
differentiate(y,x)
y = x^9
differentiate(y,x)
y = Sin(x)
differentiate(y,x)
y = Tan(x)
differentiate(y,x)
y = Cos(x)
differentiate(y,x)
y = Log(x)
differentiate(y,x)
% symbolic packages does not have this support
%y = Log10(x)
%differentiate(y,x)
y = Sin(x)^2
differentiate(y,x)
y = Cos(3*x^2 + 2*x + 1)
differentiate(y,x)
y = Exp(x)/Sin(x)
differentiate(y,x)

Octave执行代码并返回以下结果 -

y =
exp(x)
ans =
exp(x)
y =
x^(9.0)
ans =
(9.0)*x^(8.0)
y =
sin(x)
ans =
cos(x)
y =
tan(x)
ans =
1+tan(x)^2
y =
cos(x)
ans =
-sin(x)
y =
log(x)
ans =
x^(-1)
y =
sin(x)^(2.0)
ans =
(2.0)*sin(x)*cos(x)
y =
cos(1.0+(2.0)*x+(3.0)*x^(2.0))
ans =
-(2.0+(6.0)*x)*sin(1.0+(2.0)*x+(3.0)*x^(2.0))
y =
sin(x)^(-1)*exp(x)
ans =
sin(x)^(-1)*exp(x)-sin(x)^(-2)*cos(x)*exp(x)

计算高阶导数

为了计算函数f的更高导数，我们使用语法diff(f,n) 。

让我们计算函数y = f（x）= x .e ^{-3x的二阶导数}

f = x*exp(-3*x);
diff(f, 2)

MATLAB执行代码并返回以下结果 -

ans =
9*x*exp(-3*x) - 6*exp(-3*x)

以下是Octave相当于上面的计算 -

pkg load symbolic
symbols
x = sym("x");
f = x*Exp(-3*x);
differentiate(f, x, 2)

Octave执行代码并返回以下结果 -

ans =
(9.0)*exp(-(3.0)*x)*x-(6.0)*exp(-(3.0)*x)

例子 (Example)

在这个例子中，让我们解决一个问题。 假设函数y = f(x) = 3 sin(x) + 7 cos(5x) 。 我们必须弄清楚等式f" + f = -5cos(2x)成立。

创建一个脚本文件并在其中键入以下代码 -

syms x
y = 3*sin(x)+7*cos(5*x);  % defining the function
lhs = diff(y,2)+y;        %evaluting the lhs of the equation
rhs = -5*cos(2*x);        %rhs of the equation
if(isequal(lhs,rhs))
   disp('Yes, the equation holds true');
else
   disp('No, the equation does not hold true');
end
disp('Value of LHS is: '), disp(lhs);

运行该文件时，它显示以下结果 -

No, the equation does not hold true
Value of LHS is: 
-168*cos(5*x)

以下是Octave相当于上面的计算 -

pkg load symbolic
symbols
x = sym("x");
y = 3*Sin(x)+7*Cos(5*x);           % defining the function
lhs = differentiate(y, x, 2) + y;  %evaluting the lhs of the equation
rhs = -5*Cos(2*x);                 %rhs of the equation
if(lhs == rhs)
   disp('Yes, the equation holds true');
else
   disp('No, the equation does not hold true');
end
disp('Value of LHS is: '), disp(lhs);

Octave执行代码并返回以下结果 -

No, the equation does not hold true
Value of LHS is: 
-(168.0)*cos((5.0)*x)

找到曲线的最大值和最小值

如果我们正在搜索图的局部最大值和最小值，我们基本上是在特定位置的函数图上寻找最高点或最低点，或者是符号变量的特定值范围。

对于函数y = f（x），图形上具有零斜率的图上的stationary points称为stationary points 。 换句话说，静止点是f'（x）= 0的位置。

为了找到我们区分的函数的平稳点，我们需要将导数设置为零并求解方程。

例子 (Example)

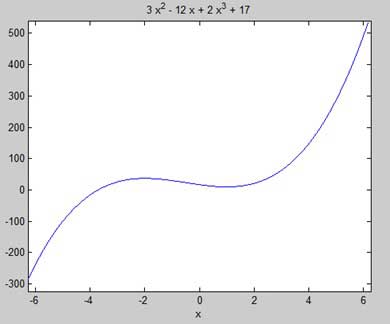
让我们找到函数f（x）= 2x ³ + 3x ² - 12x + 17的平稳点

采取以下步骤 -

First let us enter the function and plot its graph.

syms x
y = 2*x^3 + 3*x^2 - 12*x + 17;   % defining the function
ezplot(y)

MATLAB执行代码并返回以下图表 -

以下是上述示例的Octave等效代码 -

pkg load symbolic
symbols
x = sym('x');
y = inline("2*x^3 + 3*x^2 - 12*x + 17");
ezplot(y)
print -deps graph.eps

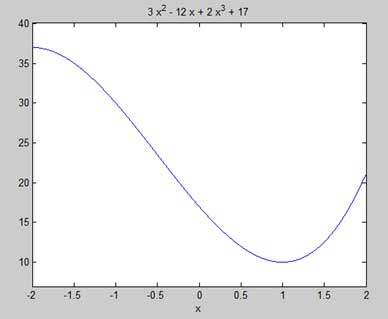
Our aim is to find some local maxima and minima on the graph, so let us find the local maxima and minima for the interval [-2, 2] on the graph.

syms x
y = 2*x^3 + 3*x^2 - 12*x + 17;   % defining the function
ezplot(y, [-2, 2])

MATLAB执行代码并返回以下图表 -

以下是上述示例的Octave等效代码 -

pkg load symbolic
symbols
x = sym('x');
y = inline("2*x^3 + 3*x^2 - 12*x + 17");
ezplot(y, [-2, 2])
print -deps graph.eps

Next, let us compute the derivative.

g = diff(y)

MATLAB执行代码并返回以下结果 -

g =
   6*x^2 + 6*x - 12

这是Octave相当于上面的计算 -

pkg load symbolic
symbols
x = sym("x");
y = 2*x^3 + 3*x^2 - 12*x + 17;
g = differentiate(y,x)

Octave执行代码并返回以下结果 -

g =
   -12.0+(6.0)*x+(6.0)*x^(2.0)

Let us solve the derivative function, g, to get the values where it becomes zero.

s = solve(g)

MATLAB执行代码并返回以下结果 -

s =
   1
   -2

以下是Octave相当于上面的计算 -

pkg load symbolic
symbols
x = sym("x");
y = 2*x^3 + 3*x^2 - 12*x + 17;
g = differentiate(y,x)
roots([6, 6, -12])

Octave执行代码并返回以下结果 -

g =
-12.0+(6.0)*x^(2.0)+(6.0)*x
ans =
  -2
   1

This agrees with our plot. So let us evaluate the function f at the critical points x = 1, -2. 我们可以使用subs命令替换符号函数中的值。

subs(y, 1), subs(y, -2)

MATLAB执行代码并返回以下结果 -

ans =
   10
ans =
   37

以下是Octave相当于上面的计算 -

pkg load symbolic
symbols
x = sym("x");
y = 2*x^3 + 3*x^2 - 12*x + 17;
g = differentiate(y,x)
roots([6, 6, -12])
subs(y, x, 1), subs(y, x, -2)

ans =
   10.0
ans =
   37.0-4.6734207789940138748E-18*I

因此，函数f（x）= 2x ³ + 3x ² - 12x + 17的最小值和最大值在区间[-2,2]中是10和37。

求解微分方程

MATLAB提供了用于象征性地求解微分方程的dsolve命令。

用于查找单个方程的解的dsolve命令的最基本形式是

dsolve('eqn') 

其中eqn是用于输入eqn的文本字符串。

它返回一个符号解决方案，其中包含一组MATLAB标记C1，C2等的任意常量。

您还可以指定问题的初始条件和边界条件，如下所示，以逗号分隔的列表为 -

dsolve('eqn','cond1', 'cond2',…)  

出于使用dsolve命令的目的，使用derivatives are indicated with a D 。 例如，输入f'（t）= -2 * f + cost（t）之类的等式作为 -

'Df = -2*f + cos(t)'

通过D导数的顺序表示更高的导数。

例如，方程f“（x）+ 2f'（x）= 5sin3x应输入 -

'D2y + 2Dy = 5*sin(3*x)'

让我们举一个简单的一阶微分方程的例子:y'= 5y。

s = dsolve('Dy = 5*y')

MATLAB执行代码并返回以下结果 -

s =
   C2*exp(5*t)

让我们将二阶微分方程的另一个例子作为:y“ - y = 0，y（0）= -1，y'（0）= 2。

dsolve('D2y - y = 0','y(0) = -1','Dy(0) = 2')

MATLAB执行代码并返回以下结果 -

ans =
   exp(t)/2 - (3*exp(-t))/2