Question

集成处理两种本质上不同类型的问题。

• 在第一种类型中，给出了函数的导数，我们想要找到函数。 因此，我们基本上扭转了分化的过程。 这种逆过程被称为反微分，或找到原始函数，或找到indefinite integral 。

• 第二类问题涉及累加非常大量的非常小的数量，然后随着数量的大小接近零而采取限制，而术语的数量倾向于无穷大。 这个过程导致了definite integral的定义。

定义积分用于寻找面积，体积，重心，转动惯量，由力完成的工作以及许多其他应用。

使用MATLAB查找不定积分

根据定义，如果函数f（x）的导数是f'（x），那么我们说f'（x）相对于x的不定积分是f（x）。 例如，由于x ²的导数（相对于x）是2x，我们可以说2x的不定积分是x ² 。

符号 -

f'(x ² ) = 2x ，因此，

∫ 2xdx = x ² .

不定积分不是唯一的，因为对于任何常数c的值，x ² + c的导数也将是2x。

这用符号表示为 -

∫ 2xdx = x ² + c 。

其中，c称为“任意常数”。

MATLAB提供了一个用于计算表达式积分的int命令。 为了得到一个函数的不定积分的表达式，我们写 -

int(f);

例如，从我们之前的例子 -

syms x 
int(2*x)

MATLAB执行上述语句并返回以下结果 -

ans =
   x^2

例子1 (Example 1)

在这个例子中，让我们找到一些常用表达式的积分。 创建一个脚本文件并在其中键入以下代码 -

syms x n
int(sym(x^n))
f = 'sin(n*t)'
int(sym(f))
syms a t
int(a*cos(pi*t))
int(a^x)

运行该文件时，它显示以下结果 -

ans =
   piecewise([n == -1, log(x)], [n ~= -1, x^(n + 1)/(n + 1)])
f =
sin(n*t)
ans =
   -cos(n*t)/n
   ans =
   (a*sin(pi*t))/pi
   ans =
   a^x/log(a)

例子2 (Example 2)

创建一个脚本文件并在其中键入以下代码 -

syms x n
int(cos(x))
int(exp(x))
int(log(x))
int(x^-1)
int(x^5*cos(5*x))
pretty(int(x^5*cos(5*x)))
int(x^-5)
int(sec(x)^2)
pretty(int(1 - 10*x + 9 * x^2))
int((3 + 5*x -6*x^2 - 7*x^3)/2*x^2)
pretty(int((3 + 5*x -6*x^2 - 7*x^3)/2*x^2))

请注意， pretty函数以更易读的格式返回表达式。

运行该文件时，它显示以下结果 -

ans =
   sin(x)
ans =
   exp(x)
ans =
   x*(log(x) - 1)
ans =
   log(x)
ans =
(24*cos(5*x))/3125 + (24*x*sin(5*x))/625 - (12*x^2*cos(5*x))/125 + (x^4*cos(5*x))/5 - (4*x^3*sin(5*x))/25 + (x^5*sin(5*x))/5
                                    2             4 
   24 cos(5 x)   24 x sin(5 x)   12 x  cos(5 x)   x  cos(5 x) 
   ----------- + ------------- - -------------- + ------------ 
      3125            625             125              5 
        3             5 
   4 x  sin(5 x)   x  sin(5 x) 
   ------------- + ----------- 
         25              5
ans =
-1/(4*x^4)
ans =
tan(x)
        2 
  x (3 x  - 5 x + 1)
ans = 
- (7*x^6)/12 - (3*x^5)/5 + (5*x^4)/8 + x^3/2
      6      5      4    3 
    7 x    3 x    5 x    x 
  - ---- - ---- + ---- + -- 
     12     5      8     2

使用MATLAB查找定积分

根据定义，定积分基本上是总和的极限。 我们使用定积分来找到诸如曲线和x轴之间的区域以及两条曲线之间的区域之类的区域。 定义积分也可用于其他情况，其中所需数量可表示为总和的极限。

通过传递要计算积分的限制， int函数可用于定积分。

计算

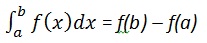
我们写，

int(x, a, b)

例如，要计算值 我们写 -

int(x, 4, 9)

MATLAB执行上述语句并返回以下结果 -

ans =
   65/2

以下是Octave相当于上面的计算 -

pkg load symbolic
symbols
x = sym("x");
f = x;
c = [1, 0];
integral = polyint(c);
a = polyval(integral, 9) - polyval(integral, 4);
display('Area: '), disp(double(a));

Octave执行代码并返回以下结果 -

Area: 
   32.500

可以使用Octave提供的quad（）函数给出替代解决方案，如下所示 -

pkg load symbolic
symbols
f = inline("x");
[a, ierror, nfneval] = quad(f, 4, 9);
display('Area: '), disp(double(a));

Octave执行代码并返回以下结果 -

Area: 
   32.500

例子1 (Example 1)

让我们计算在x轴和曲线y = x ³ -2x + 5之间的区域，并且纵坐标x = 1和x = 2。

所需面积由 - 给出 -

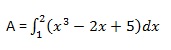
创建一个脚本文件并键入以下代码 -

f = x^3 - 2*x +5;
a = int(f, 1, 2)
display('Area: '), disp(double(a));

运行该文件时，它显示以下结果 -

a =
23/4
Area: 
   5.7500

以下是Octave相当于上面的计算 -

pkg load symbolic
symbols
x = sym("x");
f = x^3 - 2*x +5;
c = [1, 0, -2, 5];
integral = polyint(c);
a = polyval(integral, 2) - polyval(integral, 1);
display('Area: '), disp(double(a));

Octave执行代码并返回以下结果 -

Area: 
   5.7500

可以使用Octave提供的quad（）函数给出替代解决方案，如下所示 -

pkg load symbolic
symbols
x = sym("x");
f = inline("x^3 - 2*x +5");
[a, ierror, nfneval] = quad(f, 1, 2);
display('Area: '), disp(double(a));

Octave执行代码并返回以下结果 -

Area: 
   5.7500

例子2 (Example 2)

求出曲​​线下面积:f（x）= x ² cos（x）-4≤x≤9。

创建一个脚本文件并编写以下代码 -

f = x^2*cos(x);
ezplot(f, [-4,9])
a = int(f, -4, 9)
disp('Area: '), disp(double(a));

运行该文件时，MATLAB绘制图形 -

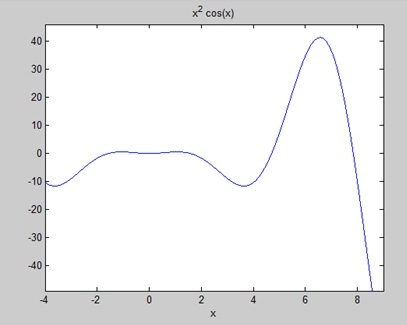
输出如下 -

a = 
8*cos(4) + 18*cos(9) + 14*sin(4) + 79*sin(9)
Area: 
   0.3326

以下是Octave相当于上面的计算 -

pkg load symbolic
symbols
x = sym("x");
f = inline("x^2*cos(x)");
ezplot(f, [-4,9])
print -deps graph.eps
[a, ierror, nfneval] = quad(f, -4, 9);
display('Area: '), disp(double(a));